算子代数与算子理论工作会议

会议地点：Zoom ID:941 567 2172 密码：110107

会议组委会: 龚贵华，纪友清，李春光，张远航，周婷婷，朱森.

                       

                        日  程 安 排

11.1 星期日	11.2 星期一	11.3 星期二	11.4 星期三	11.5 星期四	11.6 星期五	11.7 星期六
9:30-10:20	9:30-10:20	9:30-10:10	9:30-10:10	9:30-10:10	9:30-10:10	9:30-10:20
Elliott George	Lin Huaxin	Niu Zhuang	Niu Zhuang	Niu Zhuang	安庆楠	蒋春澜
10:30-11:10	10:30-11:10	10:20-11:00	10:20-11:00	10:20-11:00	10:20-11:00
Niu Zhuang	Wang Kun	Wang Kun	Wang Kun	Wang Kun	刘智超
2:00-2:40	2:00-2:40	2:00-2:40	2:00-2:40	2:00-2:40	2:00-2:40
李春光	姚一隽	李建奎	侯绳照	曹广福	侯成军
2:45-3:25	2:45-3:25	2:45-3:25	2:45-3:25	2:45-3:25	2:45-3:25
崔博	王凯	武俊德	吴文明	王茂发	王利广
	3:30-4:10	3:30-4:10
	程国正	纪奎

短课程（Short Courses）

报告题目: C*-algebras, dimensions, and dynamical systems.

报 告 人:  Zhuang Niu

报告时间: 2020年11月1日 星期日 上午10:30-11:10;

2020年11月3日 星期二 上午  9:30-10:10;

2020年11月4日 星期三 上午  9:30-10:10;

   2020年11月5日 星期四 上午  9:30-10:10.

报告摘要: I will first briefly review the classification of C*-algebras, in particular on the regularity properties and non-commutative dimensions of a C*- algebra. Then, I will focus on its applications to the structure of C*- algebras from topological dynamical systems. Only basic knowledges on C*-algebras and dynamical systems are assumed.

报告人简介:

牛壮，美国怀俄明大学教授. 2005年毕业于多伦多大学，获得博士学位. 主要研究算子代数，K理论和动力系统.在J. Reine Angew. Math., Duke Math. J., Adv. Math, J. Funct. Anal.等杂志发表多篇文章.因其在算子代数领域的卓越贡献，获得2015年加拿大算子代数的Israel Halperin奖(此奖五年一度，有时空缺)。

报告题目: C*代数分类及不变量的相关研究

报 告 人: 王琨

报告时间:  2020年11月2日 星期一 上午10:30-11:10;

2020年11月3日 星期二 上午10:20-11:00;

2020年11月4日 星期三 上午10:20-11:00;

2020年11月5日 星期四 上午10:20-11:00.

报告摘要:

在我的四个报告中，我会回顾一些单的和实秩零的C*代数的分类结果，并引入理想性质。我还会展示C*代数的不变量之间的一些关系，比如Elliott不变量，Stevens-Jiang不变量以及Hausdorffifized K1群等。最后我会讲一些关于具有理想性质的AH代数的分类结果。

报告人简介:

王琨：美国波多黎各大学博士，曾在美国德州农工大学博士后，长期从事C*代数分类以及分类的不变量的研究。主要工作有具有理想性质的分裂区间代数的分类问题，C*-代数的exponential length的控制，Elliott不变量和Stevens不变量的关系，Cuntz半群的导出等。

会议报告

报告题目: Title: How many stars in the sky?---How many C*-algebras?

报 告 人: George A. Elliott

报告时间: 2020年11月1日 星期日 上午9:30-10:20

报告摘要: Abstract. When I first gave a talk with this title twenty-five years ago, it was on the occasion of receiving the CRM-Fields-PIMS Prize for beginning to answer this question. The purpose of the present lecture is to state the final answer---in the context of simple separable amenable C*-algebras, satisfying the UCT (perhaps automatic), and well-behaved in the sense of satisfying the equivalent criteria of the Toms-Winter conjecture.

     In  other words: "This many!"

    This answer summmarizes perhaps two thousand pages of work during well over fifty years (one might say, seventy-five years) by over a hundred contributors. It is perhaps close to being as imposing a body of work as that associated with the the Hubble and (its replacement) the James Webb space telescopes.

报告人简介:

George A. Elliott教授: 是C*-代数分类理论的开创者,是国际算子代数及其应用领域的领袖人物.他提出了著名的“Elliott分类纲领”（也称为“Elliott猜想”）,数十年来引领和极大地推动了C*-代数理论的发展.1982年,George A. Elliott教授当选加拿大皇家科学院士;

1994年，被邀请在苏黎世国际数学家大会（ICM）上做报告； 1996年获得CRM-Fields Institute奖;1998年获得 Jeffery–Williams奖；1999年获得 John L. Synge Award奖；2008年，当选丹麦皇家科学院外籍院士；2012年，当选美国数学会首届会士。

报告题目: 叉积C*代数的稳定秩

报 告 人: 李春光

报告时间: 2020年11月1日 星期日 下午 2:00-2:40.

报告摘要: I will introduce the definitions of stable ranks(Bass stable rank and topological stable rank).Then I will talk about some work on the stable rank of $C^*$-algebras.  Consider a free and minimal action of $\mathbb{Z}^d$ on a compact Hausdorff space $X$. The main result shows that the stable rank

of the corresponding crossed product $C^*$-algebra $C(X)\rtimes \mathbb{Z}^d$ is $1$. This gives a positive answer to a conjecture of Niu and Phillips. This talk is based on a joint work with Zhuang Niu.

报告人简介:

李春光，东北师范大学数学与统计学院副教授。2012年毕业于必威betway，获得博士学位。主要研究算子理论与算子代数方面问题，在Trans. Amer. Math. Soc., J. Noncommut. Geom. ,Proc. Amer. Math. Soc.等杂志发表多篇文章。

报告题目: Stiefel流形上的一个可缩区域及其收缩长度的估计

报 告 人: 崔博

报告时间: 2020年11月1日 星期日 下午 2:45-3:25

报告摘要:

通过极分解将矩阵空间上到单点的收缩投射到Stiefel流形上，对退化部分进行分析，确定其是一组由秩控制的流形的不交并，且计算了最大余维数。进而分析投射到Stiefel流形上的路径长度及相应的改进，并作出长度估计。

报告人简介:

崔博，现为河北师范大学必威博士后，博士毕业于波多黎各大学。研究方向为泛函分析与算子代数。主要研究兴趣包括：K理论，C*-代数分类理论及Morse理论在C*-代数中应用。已在Journal of Topology and Analysis杂志上发表学术论文1篇。

报告题目:  Title:  Separable simple amenable stably projectionless C*-algebras

报 告 人: 林华新

报告时间: 2020年11月2日 星期一 上午 9:30-10:20

报告摘要:

We will report a unified classification for separable finite

simple amenable Jiang-Su stable C*-algebras in the UCT class.  

These C*-algebras are classified by the Elliott invariant.

We will, in particular, discuss the Elliott invariant and a reduction

theorem.

报告人简介:

林华新，华东师范大学特聘教授。1986年获得美国Purdue大学获博士学位，美国Oregon大学数学系教授，于2000年起受聘为华东师范大学紫江讲座教授，林华新教授是国际算子代数的领袖之一，是美国数学会首届会士，主要研究算子代数及其分类。  

在  Ann. of Math., Invent. Math.,    J. Reine Angew. Math.            Duke Math. J. 等顶级杂志发表多篇文章。

报告题目: Hadamard流形的拟等距性质的几何推论(根据Benoist, Hulin, Markovic等人的结果)

报 告 人: 姚一隽

报告时间: 2020年11月2日 星期一 下午 2:00-2:40

报告摘要:

我们将介绍Benoist-Hulin关于Hadamard流形的拟等距(又称粗嵌入)性质的几何推论的工作。

报告人简介:

姚一隽: 复旦大学数学科学学院教授，博士生导师。国家自然科学基金优秀青年基金获得者。主要从事泛函分析与算子代数的研究，

在Adv. Math.、J. Funct. Anal.、J. Noncommut. Geom.、Math. Z.发表多篇论文。

报告题目: Rigidity of the determinantal point processes

报 告 人: 王凯

报告时间: 2020年11月2日 星期一 下午 2:45-3:25

报告摘要:

In sharp contrast to the classical Bergman kernels, we show the existence of DPP's associated with infinite rank lacunary Bergman kernels which satisfy the Ghosh-Peres number rigidity. This is a joint work with prof. Yanqi Qiu

报告人简介:

王凯：复旦大学数学科学学院教授，博士生导师，国家自然科学基金优秀青年基金获得者，主要从事算子理论与算子代数、随机分析点过程的研究。在Hilbert模的本质正规性和不变量计算方面取得了杰出成果，代表性成果发表于Math. Ann., JFA,  IMRN等数学权威期刊。

报告题目: A Littlewood-Type Theorem on  Bergman Spaces

报 告 人: 程国正

报告时间: 2020年11月2日 星期一 下午 3:30-4:10

报告摘要:

In this talk, we consider the classical Littlewood's theorem on Bergman spaces. In detail, let

$$(\mathcal{R}f)(z)=\sum_{n=0}^{\infty}\pm  a_nz^n,\quad f(z)=\sum_{n=0}^{\infty}a_nz^n\in H(\mathbb{D}).$$

Firstly,   the space $\{f\in H(\mathbb{D}):\mathbb{P} {\mathcal{R}f\in L_a^p\}=1\}$ is characterized  entirely.  Moreover, we completely  determine the $pq$-graph of $\mathcal{R}: L_a^p\rightarrow L_a^q.$

报告人简介:

程国正：大连理工大学教授 ，博士生导师，美国数学会《数学评论》评论员。主要从事泛函分析及与随机分析的交叉研究。在包括

J. Funct. Anal.,  TAMS 等国际顶尖SCI杂志发表论文十余篇。并获得多项国家自然科学基金项目资助。

报告题目: Automatic continuity of some mappings on Banach algebras

报 告 人: 李建奎

报告时间: 2020年11月3日 星期二 下午2:00-2:40

报告摘要:

In this talk,  we report some results on automatic continuity and answer a question of Essaleh and Peralta in Linear Algebra Appl.

538 (2018), 1–21.

This is a joint with Shan Li and Kaija Luo.

报告人简介:

李建奎: 华东理工大学教授，博士生导师，现任华东理工大学理学院经理。主要从事算子代数和算子理论的研究。主持完成华东理工大学科研启动基金一项，归国留学基金一项，国家自然科学基金三项，在研国家自然科学基金一项。2014年获宝钢优秀教师奖。

报告题目: 泛线性分析简介

报 告 人: 武俊德

报告时间: 2020年11月3日 星期二 下午2:45-3:25

报告摘要:

在这个报告里，我将介绍通过改进泛函分析中著名的一致有界原理，开映射定理，闭图像定理，对偶理论，等度连续定理等，可以建立基于这些改进的基本定理的泛函分析理论，简称为泛线性分析。特别是可建立泛线性广义函数理论等。

报告人简介:

武俊德，浙江大学教授，博士生导师。主要从事泛函分析，量子信息、量子理论的数学基础相关的研究工作。在中国科学、数学物理杂志等刊物发表 109余篇研究论文，其中 80 多篇是（SCI）检索系列。多次主持国家自然科学基金等项目。

报告题目: 关于算子在不变子空间上的限制的相似性问题。

报 告 人: 纪奎

报告时间: 2020年11月3日 星期二 下午3:30-4:10

报告摘要: Bergman 空间上乘法算子在不同不变子空间上的限制何时是相似等价的是朱克和教授提出一个公开问题。在这个报告中，我们给出强不可约算子理论与Cowen-Douglas理论在此问题以及相关拓展问题的一些应用，即从复几何的角度给出关于此类相似问题的一个充分条件。

报告人简介:

纪奎，河北师范大学教授，博士生导师 ，河北师范大学数学科学院副经理。入选全国百篇优秀博士学位论文，国家自然科学基金优秀青年基金项目获得者。在Adv. Math., JFA等著名杂志发表系列优秀成果。

报告题目: Fock空间上一类积分算子的有界性

报 告 人: 侯绳照

报告时间: 2020年11月4日 星期三 下午 2:00-2:40

报告摘要:

Fock空间上的任一有界线性算子可以表示为形如。本报告将介绍Fock空间上积分算子有界性研究方面的一些进展。特别是当是朱算子或系数乘子的情形。

报告人简介:

侯绳照，苏州大学教授，主要研究函数空间上的算子理论，该领域属于复分析和泛函分析的交叉学科，有深刻的调和分析、小波分析、量子力学背景。主持完成一项国家自然科学基金项目和一项省自然科学基金项目。在国内外重要学术刊物发表论文十余篇。曾应邀赴美国、日本、意大利进行学术访问。

报告题目: Generalizations of Wigner’s Theorem

报 告 人: 吴文明

报告时间: 2020年11月4日 星期三 下午 2:45-3:25

报告摘要:

经典的Wigner定理指出保持秩1投影之间距离的满射是由一个酉算子或反-酉算子所导出的。近年来，Wigner定理得到了多种形式的推广。报告将介绍关于Wigner定理推广的主要进展、我们团队的研究结果以及一些仍有待解决的问题。

报告人简介:

吴文明：重庆师范大学教授，重庆市巴渝学者特聘教授，电子科技大学博士生导师，重庆市数学会常务理事，2012.02-2012.07访问美国新罕布什尔大学，美国《数学评论》和德国《数学文摘》特约评论员，主要研究算子代数。主持国家自然科学基金面上项目2项、省部级项目4项，在J. Funct. Anal.、Bull. Lond. Math. Soc.、Sci. China. Math.等期刊发表论文近20篇。

报告题目: Title:Boundedness of  integral operators on the fractional Fock-Sobolev spaces

报 告 人: 曹广福

报告时间: 2020年11月5日 星期四下午 2:00-2:40

报告摘要: 

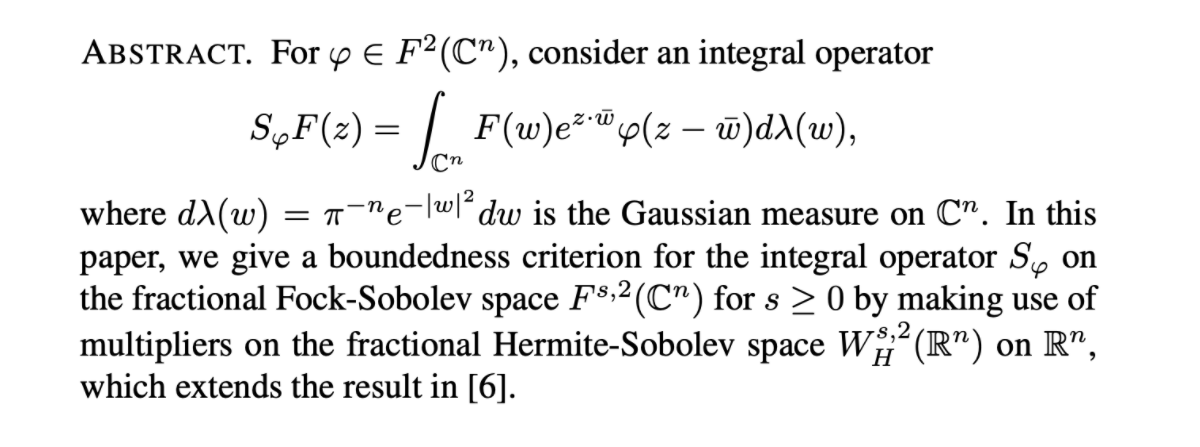
报告人简介:

曹广福，华南农业大学二级教授，博士生导师，国务院政府特殊津贴专家，宝钢优秀教师奖获得者，南粤优秀教师，四川省有突出贡献中青年专家，2003年获得国家首届高等学校教学名师奖，入选国家“万人计划”领军人才教学名师。现任教育部高等学校数学与统计学教学指导委员会专业课程教学指导分委员会委员。长期从事基础数学研究与数学教育研究，连续主持了九项国家自然科学基金与教育部高等学校博士点专项基金项目，在国内外Adv.Math、JFA、J. Geom. Anal.、 Pacific J. Math.、Nagoya Math.、 J.Tohoku Math.、J. Math. Anal. Appl.等重要刊物上发表研究论文70余篇。发表了一批数学教育研究论文，主持过教育部及省部级教改项目若干项，主编出版了“十五”、“十一五”国家规划教材，获得过国家优秀教材奖及国家基础公司产品成果奖。

报告题目: Linear combinations of composition operators with linear symbols on a Hilbert space of Dirichlet series

报 告 人: 王茂发

报告时间: 2020年11月5日 星期四 下午 2:45-3:25

报告摘要:

Motivated by classical result of linear fractional composition operators on Hardy spaces,  in this talk, we introduce that the linear combinations of two composition operators induced by linear symbols on the Hilbert space of Dirichlet series with square summable coefficients are compact only when each one of them is compact. Moreover, such rigid behavior holds partially for some more general symbols.

报告人简介:

王茂发：武汉大学数学与统计学院教授，博士生导师。主要研究方向是泛函分析及其应用，特别是函数空间上的算子理论，取得了丰硕的成果。先后多次承担863项目、国家基金委重点、面上和教育部等项目，已在J Funct Anal、J Math Anal Appl、Math Z、Indiana Uni J Math、J Operator Theory等国际著名期刊上发表学术论文40余篇。

报告题目: On the Classifcation of certain ASH-Algebras

报 告 人: 安庆楠

报告时间: 2020年11月6日 星期五 上午 9:30-10:10

报告摘要: abstract：

Point-line algebra is a concrete class of  sub-homogeneous algebras. Such algebra may have non-Riesz ordered K_0 group and arbitrary finite generated K_1group and quite a lot of interesting works are built by these algebras. We will list our resent results on the sub-homogeneous algebras，most of which are about KK-groups and classification.

报告人简介:

安庆楠, 东北师范大学数学与统计学院教师, 主要从事算子代数方面的问题研究, 相应的科研成果被数学杂志 中国科学 数学版, Journal of Operator Theory, Journal of Topology and Analysis, Mathematica Scandinavica等收录和发表.

报告题目: Distance between unitary orbits in  C*-algebras

报 告 人: 刘智超

报告时间: 2020年11月6日 星期五上午10：20—11：00

报告摘要:

One of the most fundamental questions one can ask in an operator algebra is: when are two operators unitarily equivalent? The present talk addresses the related problem of computing the distance d_U between unitary orbits in terms of spectral information. In 2008,  Elliott and Ciuperca defined two distances for any C*-algebra A that has stable rank one: one is d_U, which is defined between approximate unitary equivalence classes of *-homomorphisms from C_0(0,1] to A, and the other is d_W, defined on the semigroup of morphisms between the Cuntz semigroups, from Cu(C_0(0,1]) to Cu(A). (The distance d_W is based on the work of Weyl.) The above distances can also be regarded as  distances (pseudo-metrics) between positive elements. In this talk, we will show that the two distances are equal for C*-algebras with stable rank one and real rank zero.

报告人简介:

刘智超，大连理工大学数学科学学院副教授，主要从事C* 代数及泛函分析相关的研究工作。在研究C*代数的性质和使用 K 理论作为不变量分类C*代数方面取得一系列研究成果。在《Canad. Math. Bull.》、《J. Topol. Anal.》、《SCIENCE CHINA Mathematics》、

《Math. Scand.》等国际学术期刊发表多篇科研论文

报告题目: On C*-algebras from locally conjugate equivalence relations on expansive systems

报 告 人: 侯成军

报告时间: 2020年11月6日 星期五 下午 2:45-3:25

报告摘要:

 In this talk, we introduce notions of asymptotic continuous orbit equivalence and (strongly) asymptotic conjugacy for expansive systems, and characterize them in terms of the transformation groupoids, the principal groupoids coming from the local conjugacy relations and the semi-direct product groupoids of the principal groupoids by the canonical group actions, together with their associated reduced groupoid $C^*$-algebras. In particular, we show that two asymptotically essentially free expansive systems are  asymptotically continuous orbit equivalent if and only if the associated semi-direct product groupoids are topologically isomorphic if and only if there exists a $C^*$-algebra isomorphism preserving the canonical Cartan subalgebras between the corresponding reduced $C^*$-algebras of these semi-direct product groupoids.

报告人简介:

侯成军：扬州大学教授，博士生导师，美国数学会《Mathematics Reviews》特邀评论员；曲阜师范大学第五批中青年学术骨干。从事算子理论、算子代数及其应用的学习和研究。曾主持完成3项国家和省部级研究项目，在研一项国家自然科学基金项目; 曾获中国科学院王宽诚博士后奖励基金一项、山东省科技进步三等奖一项和山东省高校优秀科研成果奖多项。 已在 “中国科学”，“Proc. Amer. Math. Soc.”（美国）， “Bull. of Austral. Math. Soc.”（澳大利亚），“Indian J. of Pure and Applied Math.”（印度）， “Studia Mathematica”（波兰），“数学年刊”， “数学学报”，“数学物理学报”和“数学进展”等重要学术期刊发表论文20余篇；译著《泛函分析》(合作)即将由人民邮电出版社出版。

报告题目: Wigner’s theorem and $L^2$-isometry on projection lattices

报 告 人: 王利广

报告时间: 2020年11月6日 星期五 下午3:30-4:10

报告摘要:

Wigner’s theorem shows that every transition probability preserving surjection on the set of all rank one projections on a Hilbert space is induced by a unitary or an antiunitary. Wigner's theorem can be interpreted as a result of mappings that preserves certain metric on the set of projections. Recently, Geh\'{e}r and \u{S}emrl have characterized the general form of surjective isometries of the set of all projections on an infinite-dimensional separable Hilbert space using unitaries and antiunitaries. In this talk, we will study the surjective $L^2$-isometries of the projection lattice of an infinite dimensional Hilbert space and show that every such isometry can also be described by unitaries and antiunitaries. This talk is based on joint work with Wenming Wu and Wei Yuan.

报告人简介:

王利广，曲阜师范大学教授，博士生导师。2005年7月于中国科学院获理学博士学位。研究方向为泛函分析和算子代数。目前正在主持国家自然科学基金面上项目一项，主持山东省自然科学基金面上项目一项；已主持完成国家自然科学基金面上项目和数学天元基金各一项、山东省自然科学基金面上项目一项。已在J. Functional Analysis、J. Operator Theory等期刊发表论文20余篇。

报告题目: 关于Halmos第三问题的研究

报 告 人: 蒋春澜

报告时间: 2020年11月7日 星期六 上午9:30-10:30

报告摘要:

在本报告中，我们给出关于Halmos第三问题研究的最新进展。

报告人简介:

蒋春澜，现任九三学社河北省第七届委员会副主委，美国数学会会员和美国数学评论员，曾任中国科学院访问教授、美国波多黎哥大学客座教授，国家自然科学基金委员会学科评议组专家，北京大学主办《数学进展》编委、武汉大学主办《数学杂志》编委、必威betway主办的《数学通讯》编委、大连理工大学主办的《数学研究》编委，河北省数学学会理事长。长期从事算子代数可约性与强不可约性研究.在无穷维希尔伯特空间算子理论中作出了享有国际声誉的贡献，在Adv. Math.、J. Funct. Anal.、Trans. Amer. Math. Soc.等杂志发表学术论文50多篇。主持完成《国家重点基础研究发展规划》（973计划）项目、国家教育部重大课题和国家基金委重点基金项目各1项。曾获国家教委科技进步二等奖，上海市科技进步二等奖，获河北省有突出贡献的中青年专家称号。2000年获国务院特殊津贴。2003年获河北省自然科学一等奖，获教育部自然科学二等奖。